There are many more notes you can add to the basic "Root-Third-Fifth" formula. We've looked at adding the 7, which gives us major seventh chords, we also looked at adding the b7, which gives us the dominant seventh chords. The basic rule is to keep adding more odd-numbered notes above the others. By 'above', I mean higher in pitch. I know what you're thinking. You're thinking "If the scale only has seven notes in it, what could the next odd numbered note after 7 be?" The answer, logically enough, is 9. Here's how it works.

Here is a C major scale:

The scale degrees are:

Notice that I've numbered the last note '8'. It could also be numbered 1 because it's a root. Here are two octaves of the C scale now:

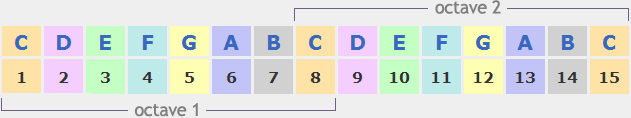
Look at how I've numbered the notes now. This is where those 'big' numbers come from. The second octave starts at 8, not 1.

The second C note is the last note of one octave but it's also the first note of the next octave. If you think of it as 8, not 1, then the next odd-numbered note is 9. If you look at the note name of the 9, which is D in this case, you'll see that D is a 2 in the first octave. 2 is an even number, 9 is an odd number. So the D has gone from being an even number to an odd number, and so have the other even numbered notes from the first octave. These notes that wind up being odd numbered notes in the next octave are called 'extensions' when added to the 1-3-5-7.

Cool? I think so ... you can see that when you get to 15, you're back to a root, which (because we're talking about chords) is there already. So there are no "15th" chords, but there are 9ths, 11ths, and 13th. There is a whole lot more to this subject than I'm attempting to explain here, but that's the basic idea. As usual, where it comes to music, there are many little twists and turns — flies in the comprehension ointment — the main one being this:

When you see a chord written like this — G11th — it is understood that the basic G chord (1-3-5) has already had the b7 added, and the 9th. So the extensions stack up on each other. Notice also that I'm saying flat 7, which means that these 'th' chords are all dominant flavor because of that b7. The plain old 7 can be used, but then the chords use the word 'major' in their names, like Gmaj9. Also, these extensions can be sharped or flatted willy-nilly, so you can get chords like G7#9, which means a G chord (1-3-5), with a b7 and a sharp 9 (#9). It's all just logical shorthand. Just remember that the numbers always refer back to the chord's root, so the 9 in a G9th chord is the ninth scale degree of the G scale. To easily figure out which note it is, subtract 7 from the larger number, so 9-7 = 2. The second note of a G scale is A.

Here's the other BIG fly in the ointment: guitars only have 6 strings and a 13th chord has 7 chord tones (1-3-5-b7-9-11-13) ... how can you play a full 13th chord on a guitar? Answer: You can't. Some notes need to be sacrificed. I won't go into any of that now except to say that the 5 is the first to go.

So what do these fancier chords look like?